Why can't a three point anchor be calculated?
RigRite works by using vector mathematics to analyze how angles affect forces in a system. There are some things that simply cannot be worked out from angles alone, three (or more) point anchor systems is one of them.
Take the following example. It’s obvious that the anchor loading is very different in these two setups; in the first the load is suspended from the outside two anchors while in the second it’s hanging straight off the centre one. You can see that knowing the angles involved is not enough to calculate the forces. Without changing the angles drastically different loading is possible.
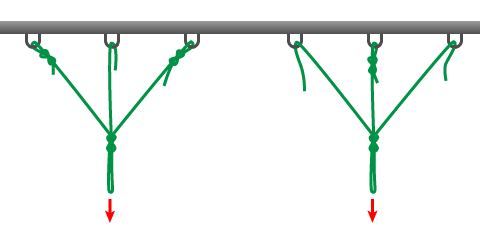
This is obviously an extreme example. In most real world examples we can assume the anchor load is roughly equal. Unfortunately ‘assume roughly equal’ is not something we can put a number on. We just don’t know how close to this extreme they really are without measuring.
What we can do
There are some things we can tell from the angles. We can calculate the range of possible forces each anchor will experience.
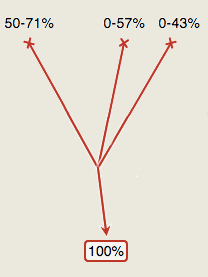
We do this by assuming there is no force on a particular anchor to see the force the other two would experience in this uneven loading situation.
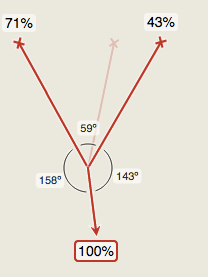
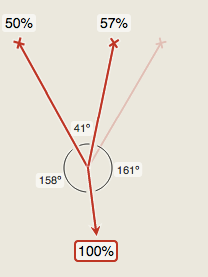
There’s no need to test with just the second and third anchor because this would require the third leg to be under compression, which isn’t possible with rope.
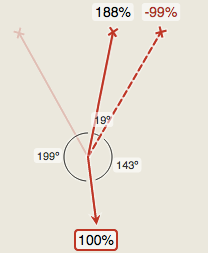
“Equal” Loading
It is only possible to have an equal force on all three anchors when the system is completely symmetrical. With asymmetrical anchors, as in the diagram above, it’s no longer possible to load the anchor equally.
There are infinite ways to distribute load within the ranges of the example above each with it’s pros and cons.
- 62% – 24% – 24% Is the most even way to share the force but the total force on the anchors is 110% of the load.
- 50% – 57% – 0% Has the lowest total force at 107% but the load is less distributed.
- 51% – 51% – 5% Minimizes the force any one anchor experiences with no anchor over 50%.


